Assessment of a new sub-grid model for magnetohydrodynamical turbulence. I. Magnetorotational instability
Abstract
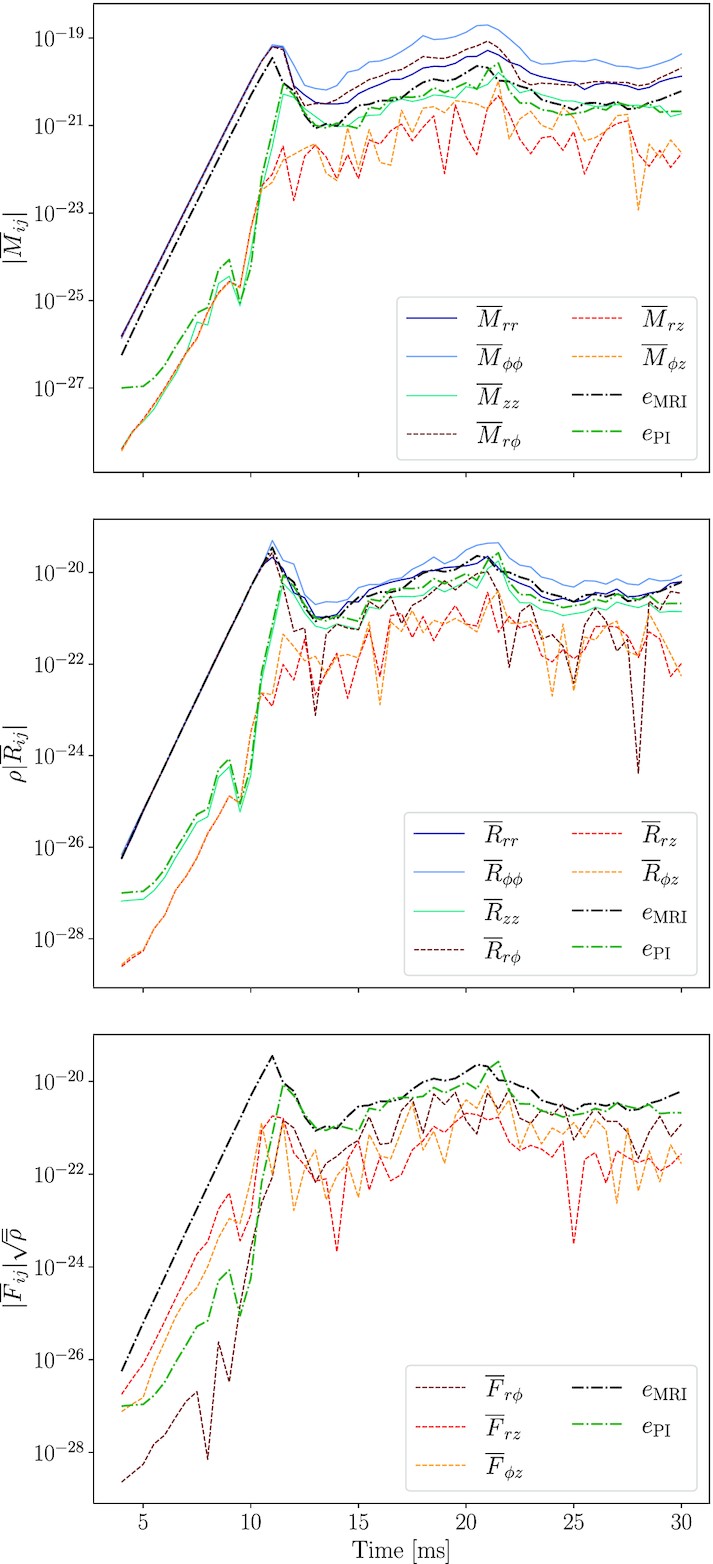
Insufficient numerical resolution of grid-based, direct numerical simulations (DNS) hampers the development of instability-driven turbulence at small (unresolved) scales. As an alternative to DNS, sub-grid models can potentially reproduce the effects of turbulence at small scales in terms of the resolved scales, and hence can capture physical effects with less computational resources. We present a new sub-grid model, the MHD-instability-induced-turbulence (MInIT) mean-field model. MInIT is a physically motivated model based on the evolution of the turbulent (Maxwell, Reynolds, and Faraday) stress tensors and their relation with the turbulent energy densities of the magnetorotational (MRI) and parasitic instabilities, modelled with two partial differential evolution equations with stiff source terms. Their solution allows obtaining the turbulent stress tensors through the constant coefficients that link them to the energy densities. The model is assessed using data from MRI in-box DNS and applying a filtering operation to compare the filtered data with that from the model. Using the L2-norm as the metric for the comparison, we find less than one order-of-magnitude difference between the two sets of data. No dependence on filter size or length scale of unresolved scales is found, as opposed to results using the gradient model (which we also use to contrast our model) in which the L2-norm of some of the stresses increases with filter size. We conclude that MInIT can help DNS by properly capturing small-scale turbulent stresses which has potential implications on the dynamics of highly magnetized rotating compact objects, such as those formed during binary neutron star mergers.
- Publication:
-
Monthly Notices of the Royal Astronomical Society
- Pub Date:
- December 2022
- DOI:
- arXiv:
- arXiv:2210.02173
- Bibcode:
- 2022MNRAS.517.3505M
- Keywords:
-
- instabilities;
- (magnetohydrodynamics) MHD;
- turbulence;
- methods: numerical;
- Astrophysics - High Energy Astrophysical Phenomena;
- Astrophysics - Solar and Stellar Astrophysics;
- Physics - Fluid Dynamics
- E-Print:
- 20 pages, 15 figures