Statistical Mechanics of Collisionless Orbits. V. The Approach to Equilibrium for Idealized Self-gravitating Systems

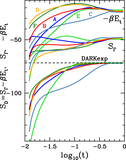
Self-gravitating Newtonian systems consisting of a very large number of particles have generally defied attempts to describe them using statistical mechanics. This is paradoxical since many astronomical systems, or simulations thereof, appear to have universal, equilibrium structures for which no physical basis exists. A decade ago we showed that extremizing the number of microstates with a given energy per unit mass, under the constraints of conserved total energy and mass, leads to the maximum entropy state, $n(E)\propto \exp (-\beta (E-{{\rm{\Phi }}}_{0}))-1$ , known as DARKexp. This differential energy distribution, and the resulting density structures, closely approximate those of dark matter halos with central cusps, ρ ~ r ^-1, and outer parts, ρ ~ r ^-4. Here we define a nonequilibrium functional, S _D , which is maximized for DARKexp and increases monotonically during the evolution toward equilibrium of idealized collisionless systems of the extended spherical infall model. Systems that undergo more mixing more closely approach DARKexp.