Stability constrained characterization of multiplanet systems
Abstract
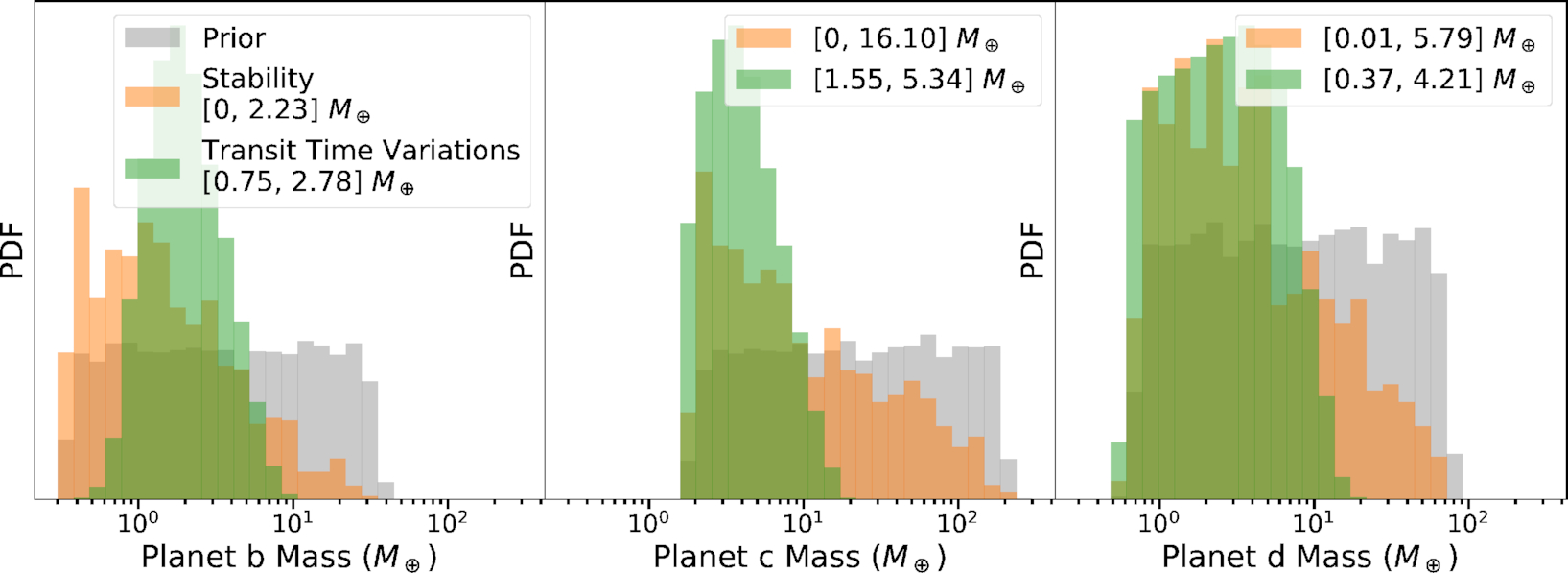
Many discovered multiplanet systems are tightly packed. This implies that wide parameter ranges in masses and orbital elements can be dynamically unstable and ruled out. We present a case study of Kepler-23, a compact three-planet system where constraints from stability, transit timing variations (TTVs), and transit durations can be directly compared. We find that in this tightly packed system, stability can place upper limits on the masses and orbital eccentricities of the bodies that are comparable to or tighter than current state of the art methods. Specifically, stability places 68 per cent upper limits on the orbital eccentricities of 0.09, 0.04, and 0.05 for planets b, c, and d, respectively. These constraints correspond to radial velocity signals ≲ 20 cm s-1, are significantly tighter to those from transit durations, and comparable to those from TTVs. Stability also yields 68 per cent upper limits on the masses of planets b, c, and d of 2.2, 16.1, and 5.8 M⊕, respectively, which were competitive with TTV constraints for the inner and outer planets. Performing this stability constrained characterization is computationally expensive with N-body integrations. We show that SPOCK, the Stability of Planetary Orbital Configurations Klassifier, is able to faithfully approximate the N-body results over 4000 times faster. We argue that such stability constrained characterization of compact systems is a challenging 'needle-in-a-haystack' problem (requiring removal of 2500 unstable configurations for every stable one for our adopted priors) and we offer several practical recommendations for such stability analyses.
- Publication:
-
Monthly Notices of the Royal Astronomical Society
- Pub Date:
- March 2021
- DOI:
- arXiv:
- arXiv:2009.11831
- Bibcode:
- 2021MNRAS.501.4798T
- Keywords:
-
- planets and satellites;
- dynamical evolution and stability;
- Astrophysics - Earth and Planetary Astrophysics
- E-Print:
- Responded to minor referee comments. Repository with notebooks to reproduce figures / for analysis of new systems at https://github.com/dtamayo/Stability-Priors