The effect of photometric redshift uncertainties on galaxy clustering and baryonic acoustic oscillations
Abstract
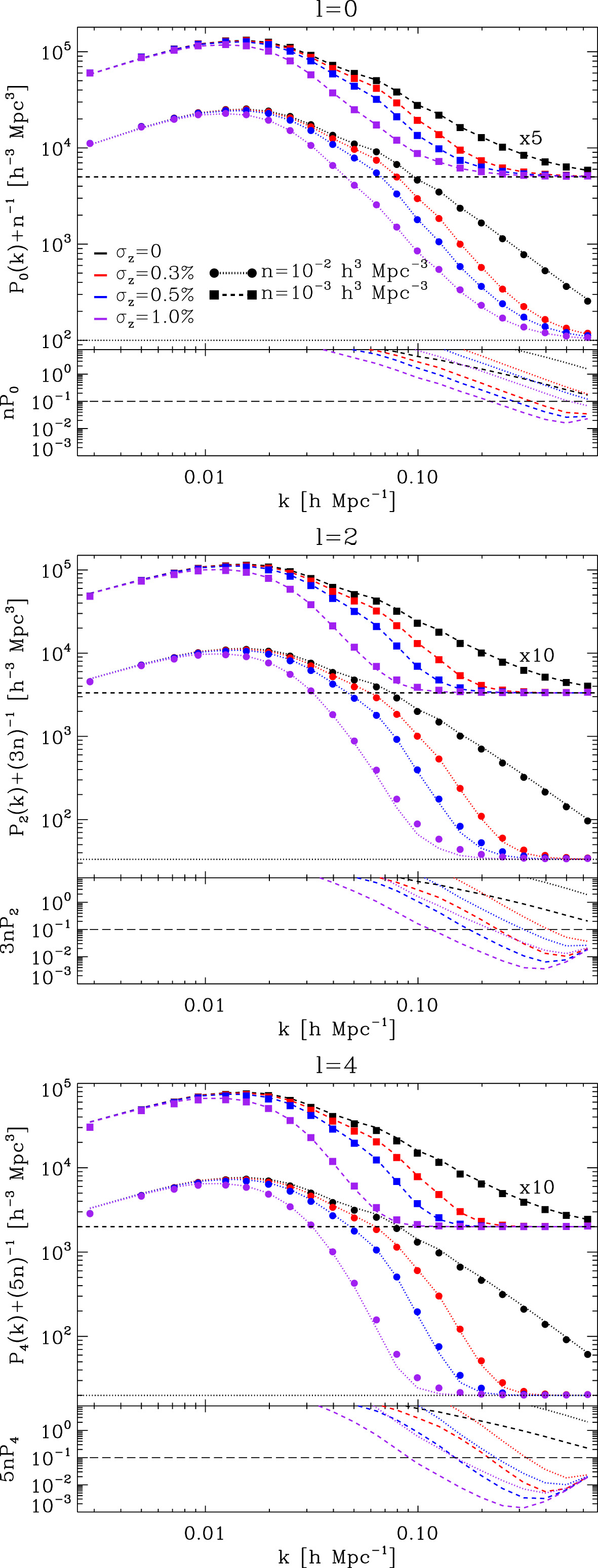
In the upcoming era of high-precision galaxy surveys, it becomes necessary to understand the impact of redshift uncertainties on cosmological observables. In this paper we explore the effect of sub-percent photometric redshift errors (photo-z errors) on galaxy clustering and baryonic acoustic oscillations (BAOs). Using analytic expressions and results from 1000 N-body simulations, we show how photo-z errors modify the amplitude of moments of the 2D power spectrum, their variances, the amplitude of BAOs, and the cosmological information in them. We find that (a) photo-z errors suppress the clustering on small scales, increasing the relative importance of shot noise, and thus reducing the interval of scales available for BAO analyses; (b) photo-z errors decrease the smearing of BAOs due to non-linear redshift-space distortions (RSDs) by giving less weight to line-of-sight modes; and (c) photo-z errors (and small-scale RSD) induce a scale dependence on the information encoded in the BAO scale, and that reduces the constraining power on the Hubble parameter. Using these findings, we propose a template that extracts unbiased cosmological information from samples with photo-z errors with respect to cases without them. Finally, we provide analytic expressions to forecast the precision in measuring the BAO scale, showing that spectro-photometric surveys will measure the expansion history of the Universe with a precision competitive to that of spectroscopic surveys.
- Publication:
-
Monthly Notices of the Royal Astronomical Society
- Pub Date:
- July 2018
- DOI:
- arXiv:
- arXiv:1610.09688
- Bibcode:
- 2018MNRAS.477.3892C
- Keywords:
-
- large-scale structure of the Universe;
- techniques: photometric;
- surveys;
- galaxies: distances and redshifts;
- cosmological parameters;
- distance scale;
- Astrophysics - Cosmology and Nongalactic Astrophysics
- E-Print:
- 19 pages, 15 figures, resubmission. Included full analysis of power spectrum moments l=0, 2, and 4