A Universal Probability Distribution Function for Weak-lensing Amplification

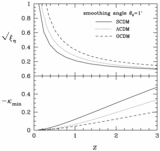
We present an approximate form for the weak-lensing magnification distribution of standard candles, valid for all cosmological models, with arbitrary matter distributions, over all redshifts. Our results are based on a universal probability distribution function (UPDF), P(η), for the reduced convergence, η. For a given cosmological model, the magnification probability distribution, P(μ), at redshift z is related to the UPDF by P(μ)=P(η)/(2|κ_min|), where η=1+(μ-1)/(2|κ_min|), and κ_min (the minimum convergence) can be directly computed from the cosmological parameters (Ω_m and Ω_Λ). We show that the UPDF can be well approximated by a three-parameter stretched Gaussian distribution, where the values of the three parameters depend only on ξ_η, the variance of η. In short, all possible weak-lensing probability distributions can be well approximated by a one-parameter family. We establish this family, normalizing to the numerical ray-shooting results for a Λ cold dark matter (CDM) model by Wambsganss et al. (1997). Each alternative cosmological model is then described by a single function ξ_η(z). We find that this method gives P(μ) in excellent agreement with numerical ray-tracing and three-dimensional shear matrix calculations, and we provide numerical fits for three representative models (SCDM, ΛCDM, and OCDM). Our results provide an easy, accurate, and efficient method to calculate the weak-lensing magnification distribution of standard candles and should be useful in the analysis of future high-redshift supernova data.