Accretion of a Satellite onto a Spherical Galaxy: Binary Evolution and Orbital Decay

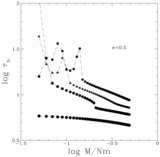
We study the dynamical evolution of a satellite (of mass M) orbiting around a companion spherical galaxy. The satellite is subject to a back-reaction force F_Δ resulting from the density fluctuations excited in the primary stellar system. We evaluate this force using the linear response theory developed by Colpi & Pallavicini in 1998. F_Δ is computed in the reference frame comoving with the primary galaxy and is expanded in multipoles. To lowest order, the force depends on a time integral involving a dynamical four-point correlation function of the unperturbed background. The equilibrium stellar system (of mass Nm) is described in terms of a Gaussian one-particle distribution function. To capture the relevant features of the physical process determining the evolution of the detached binary, we introduce in the Hamiltonian the harmonic potential as interaction potential among stars. The evolution of the composite system is derived solving for a set of ordinary differential equations; the dynamics of the satellite and of the stars is computed self-consistently. We determine the conditions for tidal capture of a satellite from an asymptotic free state and give an estimate of the maximum kinetic energy above which encounters do not end in a merger as a function of the mass ratio M/Nm. We find that capture always leads to final coalescence. If the binary forms as a bound pair, stability against orbital decay is lost if the pericentric distance is smaller than a critical value. This instability is interpreted in terms of a near-resonance condition and establishes when the orbital Keplerian frequency becomes comparable to the internal frequency ω of the stellar system. We show that before coalescence, eccentric orbits become progressively less eccentric; the circularization is explored as a function of mass ratio. The timescale of binary coalescence τ_b is a sensitive function of the eccentricity e for a fixed semimajor axis a and M/Nm ratio: the mismatch between τ_b at e ~ 0 and τ_b at e ~ 1 can be very large, typically τ_b(e <~ 1) ~ 6ω^-1, and the time ratio τ_b(0)/τ_b(1) >~ 5 (for M/Nm = 0.05). In addition, we find that τ_b obeys a scaling relation with M/Nm for circular orbits: τ_b ~ (M/Nm)^-α, with α ~ 0.4. In grazing encounters, τ_b is nearly independent of mass.