Stability Limits in Extrasolar Planetary Systems
Abstract
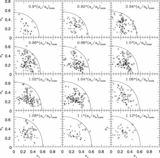
Two types of stability boundaries exist for any planetary system consisting of one star and two planets. Lagrange stability requires that the planets remain bound to the star, conserves the ordering of the distance from the star, and limits the variations of orbital elements like semimajor axis and eccentricity. Hill stability only requires that the ordering of the planets remain constant; the outer planet may escape to infinity. A simple formula defines a region in orbital element space that is guaranteed to be Hill-stable, although Hill-stable orbits may lie outside the region as well. No analytic criteria describe Lagrange stability. We compare the results of 1000 numerical simulations of planetary systems similar to 47 UMa and HD 12661 with these two types of boundaries. All cases are consistent with the analytic criterion for Hill stability. Moreover, the numerically determined Lagrange boundary lies close to the analytic boundary for Hill stability. This result suggests an analytic formulation that may describe the criterion for Lagrange stability.
- Publication:
-
The Astrophysical Journal
- Pub Date:
- August 2006
- DOI:
- arXiv:
- arXiv:astro-ph/0607210
- Bibcode:
- 2006ApJ...647L.163B
- Keywords:
-
- Methods: Analytical;
- Methods: n-Body Simulations;
- Stars: Planetary Systems;
- Stars: Individual: Henry Draper Number: HD 12661;
- Stars: Individual: Constellation Name: 47 Ursae Majoris;
- Astrophysics
- E-Print:
- 13 pages, 4 figures, accepted ApJ Letters