The Inverse Cascade and Nonlinear Alpha-Effect in Simulations of Isotropic Helical Hydromagnetic Turbulence

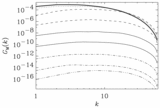
A numerical model of isotropic homogeneous turbulence with helical forcing is investigated. The resulting flow, which is essentially the prototype of the α² dynamo of mean field dynamo theory, produces strong dynamo action with an additional large-scale field on the scale of the box (at wavenumber k=1; forcing is at k=5). This large-scale field is nearly force free and exceeds the equipartition value. As the magnetic Reynolds number R_m increases, the saturation field strength and the growth rate of the dynamo increase. However, the time it takes to build up the large-scale field from equipartition to its final superequipartition value increases with magnetic Reynolds number. The large-scale field generation can be identified as being due to nonlocal interactions originating from the forcing scale, which is characteristic of the α-effect. Both α and turbulent magnetic diffusivity η_t are determined simultaneously using numerical experiments where the mean field is modified artificially. Both quantities are quenched in an R_m-dependent fashion. The evolution of the energy of the mean field matches that predicted by an α² dynamo model with similar α and η_t quenchings. For this model an analytic solution is given that matches the results of the simulations. The simulations are numerically robust in that the shape of the spectrum at large scales is unchanged when changing the resolution from 30³ to 120³ mesh points, or when increasing the magnetic Prandtl number (viscosity/magnetic diffusivity) from 1 to 100. Increasing the forcing wavenumber to 30 (i.e., increasing the scale separation) makes the inverse cascade effect more pronounced, although it remains otherwise qualitatively unchanged.