Overstable Librations can Account for the Paucity of Mean Motion Resonances among Exoplanet Pairs
Abstract
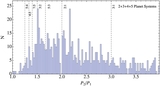
We assess the multi-planet systems discovered by the Kepler satellite in terms of current ideas about orbital migration and eccentricity damping due to planet-disk interactions. Our primary focus is on first order mean motion resonances, which we investigate analytically to lowest order in eccentricity. Only a few percent of planet pairs are in close proximity to a resonance. However, predicted migration rates (parameterized by \tau _n=n/{|\dot{n}|}) imply that during convergent migration most planets would have been captured into first order resonances. Eccentricity damping (parameterized by \tau _e=e/{|\dot{e}|}) offers a plausible resolution. Estimates suggest τ e /τ n ~ (h/a)2 ~ 10-2, where h/a is the ratio of disk thickness to radius. Together, eccentricity damping and orbital migration give rise to an equilibrium eccentricity, e eq ~ (τ e /τ n )1/2. Capture is permanent provided e eq <~ μ1/3, where μ denotes the planet to star mass ratio. But for e eq >~ μ1/3, capture is only temporary because librations around equilibrium are overstable and lead to passage through resonance on timescale τ e . Most Kepler planet pairs have e eq > μ1/3. Since τ n Gt τ e is the timescale for migration between neighboring resonances, only a modest percentage of pairs end up trapped in resonances after the disk disappears. Thus the paucity of resonances among Kepler pairs should not be taken as evidence for in situ planet formation or the disruptive effects of disk turbulence. Planet pairs close to a mean motion resonance typically exhibit period ratios 1%-2% larger than those for exact resonance. The direction of this shift undoubtedly reflects the same asymmetry that requires convergent migration for resonance capture. Permanent resonance capture at these separations from exact resonance would demand μ(τ n /τ e )1/2 >~ 0.01, a value that estimates of μ from transit data and (τ e /τ n )1/2 from theory are insufficient to match. Plausible alternatives involve eccentricity damping during or after disk dispersal. The overstability referred to above has applications beyond those considered in this investigation. It was discovered numerically by Meyer & Wisdom in their study of the tidal evolution of Saturn's satellites.
- Publication:
-
The Astronomical Journal
- Pub Date:
- February 2014
- DOI:
- arXiv:
- arXiv:1308.4688
- Bibcode:
- 2014AJ....147...32G
- Keywords:
-
- celestial mechanics;
- methods: analytical;
- methods: numerical;
- planet-disk interactions;
- planets and satellites: dynamical evolution and stability;
- planets and satellites: formation;
- Astrophysics - Earth and Planetary Astrophysics
- E-Print:
- Accepted for publication in AJ. Corrected typos, updated references, added Appendix