A two-parameter family of double-power-law biorthonormal potential-density expansions

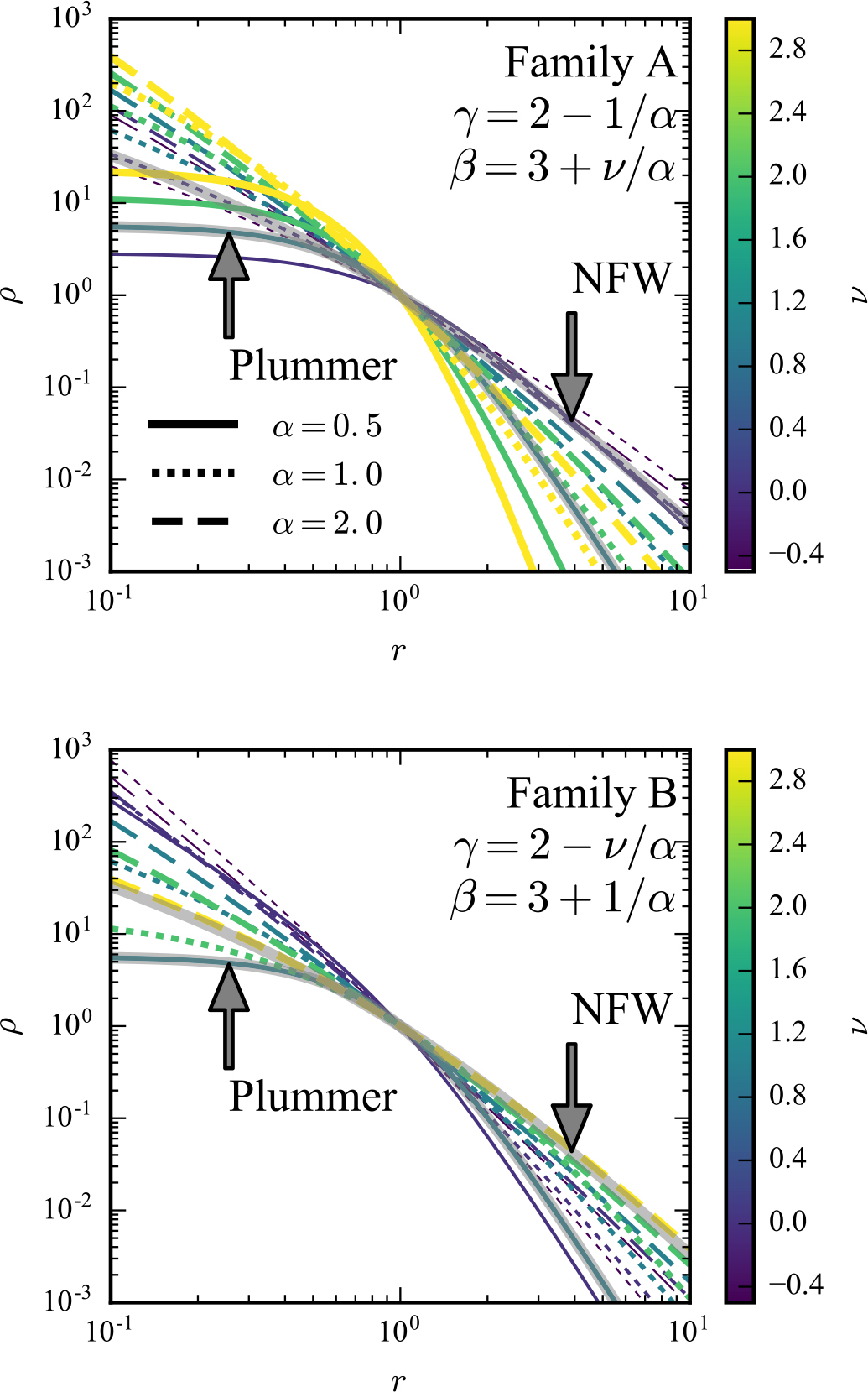
We present a two-parameter family of biorthonormal double-power-law potential-density expansions. Both the potential and density are given in a closed analytic form and may be rapidly computed via recurrence relations. We show that this family encompasses all the known analytic biorthonormal expansions: the Zhao expansions (themselves generalizations of ones found earlier by Hernquist & Ostriker and by Clutton-Brock) and the recently discovered Lilley et al. expansion. Our new two-parameter family includes expansions based around many familiar spherical density profiles as zeroth-order models, including the γ models and the Jaffe model. It also contains a basis expansion that reproduces the famous Navarro-Frenk-White (NFW) profile at zeroth order. The new basis expansions have been found via a systematic methodology which has wide applications in finding other new expansions. In the process, we also uncovered a novel integral transform solution to Poisson's equation.